Rotating Equatorial Microphone (REM)
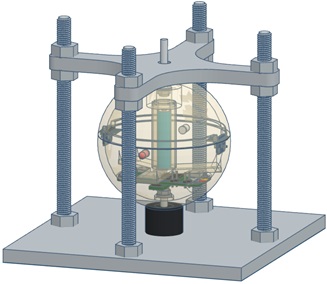
The Rotating Equatorial Microphone, or REM for short, was developed as part of a research project to enable processing methods that typically require spherical microphone arrays using only a single moving microphone. The maximum speed of the REM is at least 200 rotations per second (RPS), however, wind and motor noise heavily distort recorded audio signals, such that the maximum usable speed was found to be approximately 42 RPS. It should be noted that the true top speed of the REM is unknown since the speed was not increased beyond 200 RPS due to safety concerns. More information regarding the design of the REM can be found here. Although two microphones are included in the REM for balance reasons, only one of the microphones has been utilized so far, while the other one serves as a backup.
Here is a short demonstration video where the REM spins up to a speed of 150 RPS. The outside edge of the microphone housing moves at approximately 170 km/h at this rotational speed!
Sound Source Localization
Estimating the Direction Of Arrival (DOA) of sound sources typically requires at least two microphones, since many localization approaches rely on time difference of arrival or power difference estimates of each sound source at multiple microphones. As a consequence, the task of localizing sound sources using a single channel is inherently challenging. Multiple approaches for single channel sound source localization have been proposed, which typically utilize a scattering body placed close to the microphone, that induces direction-dependent localization cues into recorded signals. However, these approaches only work given prior knowledge of the sources to be localized, as it is otherwise impossible to distinguish between the localization cues and the sound to be localized. This problem can be circumvented by introducing microphone movement: The rotation of the REM induces DOA-dependent periodic distortions into recorded signals. These distortions mainly consist of periodic frequency shifts and periodic changes in amplitude due to the Doppler effect and the microphone's directivity. Given knowledge of the instantaneous position and the directivity of the microphone, it is possible to compensate for these distortions for multiple DOA guesses. Subsequently, the compensated signal with the least distortion can be identified and the associated DOA guess is selected as the best DOA estimate. Note that for this method to be effective, the source signals must remain relatively constant within one microphone rotation since the extent of the distortions can otherwise not be quantified. Therefore, high rotational speeds are employed to ensure that this property holds for most real-world sounds.
Consider the following example:
A sound source is situated in the far field and emits the following 2000 Hz sine wave (lower your volume levels just in case!):
This signal reaches the REM at an azimuth angle of, e.g., φ=180°. The (simulated) signal captured by the REM spinning at 24 RPS sounds as follows:
These distortions can now be compensated for multiple values of φ:
It is evident that the distortions are reduced as φ approaches the correct value. Here is another example with an excerpt from a piano concerto as the source signal:
This signal again reaches the REM at φ=180° and the (simulated) captured signal corresponds to:
The compensated signals are given below:
In practical scenarios, the recorded signals are subject to significant distortion due to wind and motor noise, particularly at higher rotational speeds. Consequently, it is necessary to employ noise reduction techniques to filter the noise from recorded signals. Below you can find the the same samples from earlier, which were recorded by the REM in a low-reverberant environment at three different rotational speeds. Additionally, the filtered counterparts of each recorded signal are also provided. The filtered versions were generated using spectral subtraction based on a 1-second noise sample.
Real-world 2000 Hz signal captured by the REM:
Real-world piano concerto excerpt captured by the REM:
As it can be observed, spectral subtraction is very effective at reducing the noise, however, some artifacts remain. Unsurprisingly, the quality of the filtered signals is also better at lower rotational speeds.
Let us now apply the same compensation from before to the filtered real-world audio signals that have been captured at a rotational speed of 24 RPS and a DOA of φ=180°.
Compensating for the recorded distortions in the 2000 Hz signal:
Compensating for the recorded distortions in the piano concerto excerpt:
It is apparent that the distortions caused by the microphone rotation significantly decrease as the angle φ approaches 180°, although some minor distortions persist. We expect that future enhancements to the distortion compensation algorithm will help mitigate this issue. Additionally, the REM prototype can be significantly improved, as it was originally designed for much higher rotational speeds. Optimizing the hardware for speeds between 20-50 RPS should result in a noticeable reduction in self-noise.
Some closing remarks on sound source localization:
We found that the presented DOA estimation approach is accurate within 5.4 degrees for unknown wideband sources. Furthermore, we have found that the same approach can be used to locate multiple sources, albeit with reduced accuracy. Finally, the distortions that are induced by the REM don't only depend on the azimuth angle of sound sources, but also their elevation. Therefore, full 3D localization is feasible using this approach. A detailed explanation and evaluation of the compensation algorithm, including an evaluation of the metrics that quantify the degree of distortion in the compensated signals, can be found here. A shortened version of the previously mentioned article can be found here and the previous iteration of this localization approach can be found here.
References (Click to Open)
@article{Lawrence_2024_Frontiers,
title = {Direction of Arrival Estimation Using the Rotating Equatorial Microphone},
author = {Jeremy Lawrence and Jens Ahrens and Nils Peters},
journal = {Frontiers in Signal Processing},
volume = {4},
year = {2024},
publisher = {Frontiers Signal Processing},
doi = {10.3389/frsip.2024.1341087},
url-demo = {https://www.audiolabs-erlangen.de/fau/assistant/lawrence/REM},
}
@INPROCEEDINGS{Lawrence_2024_DAGA,
author = {Jeremy Lawrence and Jens Ahrens and Nils Peters},
booktitle = {Fortschritte der Akustik - DAGA 2024},
title = {Direction of Arrival Estimation with a Circularly Moving Microphone},
year = {2024},
volume = {},
number = {},
pages = {949-952},
url-pdf = {https://pub.dega-akustik.de/DAGA_2024/files/upload/paper/591.pdf}}
}
@mastersthesis{Lawrence_2023,
type = {{{Master thesis}}},
title = {Sound Source Localization with the Rotating Equatorial Microphone (REM)},
author = {Jeremy Lawrence},
year = {2023},
address = {{Erlangen, Germany}},
langid = {english},
doi = {10.13140/RG.2.2.34073.19045/1},
school = {Friedrich-Alexander-Universität Erlangen-Nürnberg},
}
@INPROCEEDINGS{Lawrence_2022,
author = {Jeremy Lawrence and Jens Ahrens and Nils Peters},
booktitle = {2022 International Workshop on Acoustic Signal Enhancement (IWAENC)},
title = {Comparison of Position Estimation Methods for the Rotating Equatorial Microphone},
year = {2022},
volume = {},
number = {},
pages = {1-5},
doi = {10.1109/IWAENC53105.2022.9914776}}